문제
You are given an array points where points[i] = [xi, yi] is the coordinates of the ith point on a 2D plane. Multiple points can have the same coordinates.
You are also given an array queries where queries[j] = [xj, yj, rj] describes a circle centered at (xj, yj) with a radius of rj.
For each query queries[j], compute the number of points inside the jth circle. Points on the border of the circle are considered inside.
Return an array answer, where answer[j] is the answer to the jth query.
Example 1:
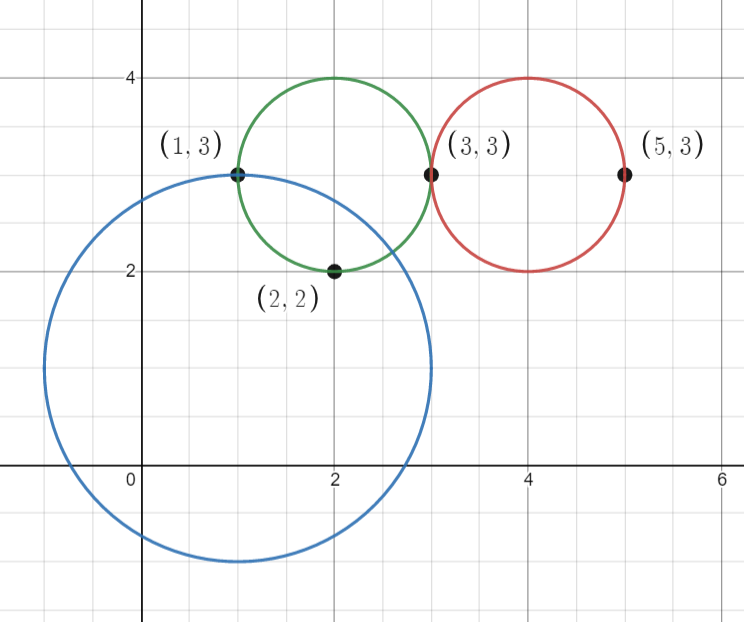
Input: points = [[1,3],[3,3],[5,3],[2,2]], queries = [[2,3,1],[4,3,1],[1,1,2]]
Output: [3,2,2]
Explanation: The points and circles are shown above.
queries[0] is the green circle, queries[1] is the red circle, and queries[2] is the blue circle.
Example 2:
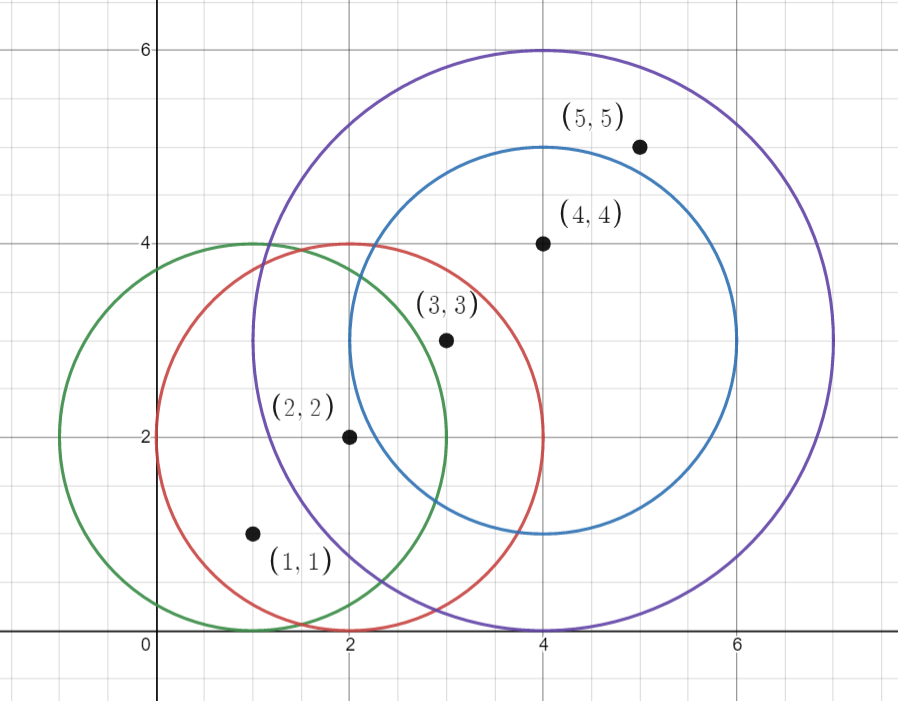
Input: points = [[1,1],[2,2],[3,3],[4,4],[5,5]], queries = [[1,2,2],[2,2,2],[4,3,2],[4,3,3]]
Output: [2,3,2,4]
Explanation: The points and circles are shown above.
queries[0] is green, queries[1] is red, queries[2] is blue, and queries[3] is purple.
Constraints:
- 1 <= points.length <= 500
- points[i].length == 2
- 0 <= xi, yi <= 500
- 1 <= queries.length <= 500
- queries[j].length == 3
- 0 <= xj, yj <= 500
- 1 <= rj <= 500
- All coordinates are integers.
먼저 answer 벡터를 만들고 queries 벡터의 행 크기만큼 반복한다.
다시 points 벡터의 행 크기만큼 반복하면서 points의 x, y 좌표가 원 안에 들어있는지 확인하여 원 안에 있는 점의 개수를 센다.
원 안에 들어있는지 확인하는 방법은 다음과 같다.
좌표평면상에 원의 중심점 (x0, y0) 가 있고 어떤 점 (x1, y1) 가 있을 때 두 점 사이의 직선 거리는 피타고라스의 정리에 의해 (x1-x0)^2 + (y1-y0)^2 를 루트씌운 것과 같은데 이게 반지름 r 보다 작거나 같다면 해당 좌표는 원 안에 있다고 판단할 수 있다.
class Solution {
public:
vector<int> countPoints(vector<vector<int>>& points, vector<vector<int>>& queries) {
vector<int> answer(queries.size());
for(int i = 0 ; i < queries.size(); i++)
{
int in_circle = 0;
for(int j =0 ; j < points.size(); j++)
{
if(pow(points[j][0] - queries[i][0], 2) + pow(points[j][1] - queries[i][1], 2) <= pow(queries[i][2], 2)) in_circle++;
}
answer[i] = in_circle;
}
return answer;
}
};
'코딩테스트' 카테고리의 다른 글
| C언어 ] leetCode 2181 - Merge Nodes in Between Zeros (0) | 2023.05.07 |
|---|---|
| C++ ] leetCode 2610 - Convert an Array Into a 2D Array With Conditions (0) | 2023.04.09 |
| C++ ] leetCode 64 - Minimum Path Sum (0) | 2023.03.31 |
| C++ ] leetCode 1476 - Subrectangle Queries (0) | 2023.03.31 |
| C언어 ] 프로그래머스 Lv.2 - 피보나치 수 (0) | 2023.03.31 |



